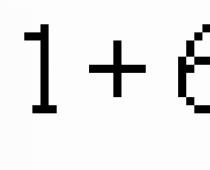The amount of motion of the system, as a vector quantity, is determined by formulas (4.12) and (4.13).
Theorem. The time derivative of the amount of motion of the system is equal to the geometric sum of all external forces acting on it.
In the projections of the Cartesian axes, we obtain scalar equations.
You can write a vector
(4.28)
and scalar equations
Which express the theorem on the change in the momentum of the system in integral form: the change in the momentum of the system over a certain period of time is equal to the sum of the impulses for the same period of time. When solving problems, equations (4.27) are more often used
Law of conservation of momentum
Theorem on the change of the kinetic moment
The theorem on the change in the moment of momentum of a point relative to the center: the time derivative of the moment of momentum of a point relative to a fixed center is equal to vector moment acting on a point of force about the same center.
or 
(4.30)
Comparing (4.23) and (4.30), we see that the moments of the vectors and are connected by the same dependence as the vectors themselves and are connected (Fig. 4.1). If we project equality onto the axis passing through the center O, we get
(4.31)
This equality expresses the theorem of the moment of momentum of a point about an axis.
|
(4.32)
If we project the expression (4.32) onto the axis passing through the center O, then we obtain an equality that characterizes the theorem on the change in the angular momentum relative to the axis.
(4.33)
Substituting (4.10) into equality (4.33) one can write down the differential equation of a rotating rigid body (wheels, axles, shafts, rotors, etc.) in three forms.
(4.34)
(4.35)
(4.36)
Thus, it is advisable to use the theorem on the change in the kinetic moment to study the motion of a rigid body, which is very common in technology, its rotation around a fixed axis.
The law of conservation of the angular momentum of the system
1. Let in expression (4.32) .
Then it follows from equation (4.32) that , i.e. if the sum of the moments of all external forces applied to the system relative to a given center is zero, then the kinetic moment of the system relative to this center will be numerically and in direction will be constant.
2. If , then . Thus, if the sum of the moments of external forces acting on the system with respect to some axis is equal to zero, then the kinetic moment of the system with respect to this axis will be a constant value.
These results express the law of conservation of angular momentum.
In the case of a rotating rigid body, it follows from equality (4.34) that if , then . From here we come to the following conclusions:
If the system is immutable (absolutely solid), then , therefore, and and the rigid body rotates around a fixed axis with a constant angular velocity.
If the system is changeable, then . With an increase (then the individual elements of the system move away from the axis of rotation), the angular velocity decreases, because , and increases with decreasing, thus, in the case of a variable system, with the help of internal forces, it is possible to change the angular velocity.
Second task D2 control work is devoted to the theorem on the change in the kinetic moment of the system about the axis.
Task D2
A homogeneous horizontal platform (round with radius R or rectangular with sides R and 2R, where R = 1.2 m) with mass kg rotates with an angular velocity around the vertical axis z, which is spaced from the center of mass C of the platform at a distance OC = b (Fig. D2.0 – D2.9, table D2); dimensions for all rectangular platforms are shown in fig. D2.0a (top view).
At the moment of time, a load D with a mass of kg begins to move along the platform chute (under the action of internal forces) according to the law , where s is expressed in meters, t is in seconds. At the same time, a pair of forces with a moment M (given in newtonometers) begin to act on the platforms; at M< 0 его направление противоположно показанному на рисунках).
Determine, neglecting the mass of the shaft, the dependence i.e. platform angular velocity as a function of time.
In all figures, the load D is shown in a position where s > 0 (when s< 0, груз находится по другую сторону от точки А). Изображая чертеж решаемой задачи, провести ось z на заданном расстоянии OC = b от центра C.
Directions. Task D2 - on the application of the theorem on the change in the angular momentum of the system. When applying the theorem to a system consisting of a platform and a load, the angular momentum of the system about the z-axis is defined as the sum of the moments of the platform and the load. In this case, it should be taken into account that the absolute speed of the load is the sum of the relative and portable speeds, i.e. . Therefore, the amount of movement of this cargo 
. Then we can use the Varignon theorem (statics), according to which ; these moments are calculated in the same way as the moments of forces. The course of the solution is explained in more detail in example D2.
When solving the problem, it is useful to depict on the auxiliary drawing a view of the platform from above (from the end z), as is done in Fig. D2.0,a - D2.9,a.
The moment of inertia of a plate with mass m about the axis Cz, perpendicular to the plate and passing through its center of mass, is equal to: for a rectangular plate with sides and
;
For a round insert of radius R
| Condition number | b | s = F(t) | M |
| R R/2 R R/2 R R/2 R R/2 R R/2 | -0.4 0.6 0.8 10t 0.4 -0.5t -0.6t 0.8t 0.4 0.5 | 4t -6 -8t -9 6 -10 12 |
|
|
|
|
|
|
|
|
|
|||
|
|||
|
|||
|
|||
|
|
|
|
|
|
|
|
|
Example D2. A homogeneous horizontal platform (rectangular with sides 2l and l), having a mass, is rigidly fastened to a vertical shaft and rotates with it around an axis z with angular velocity (Fig. E2a ). At the moment of time, a torque M begins to act on the shaft, directed oppositely ; at the same time cargo D mass located in the gutter AB at the point WITH, starts to move along the chute (under the action of internal forces) according to the law s = CD = F(t).
Given: m 1 \u003d 16 kg, t 2= 10 kg, l\u003d 0.5 m, \u003d 2, s \u003d 0.4t 2 (s - in meters, t - in seconds), M= kt, where k=6 Nm/s. Determine: - the law of change of the angular velocity of the platform.
Solution. Consider a mechanical system consisting of a platform and a load D. To determine w, we apply the theorem on the change in the angular momentum of the system about the axis z:
(1)
Let us depict the external forces acting on the system: the forces of gravity of the reaction and the torque M. Since the forces and are parallel to the z axis, and the reactions intersect this axis, their moments relative to the z axis are equal to zero. Then, considering the positive direction for the moment (i.e., counterclockwise), we obtain 
and equation (1) will take this form.
Theorem on the change in momentum of a point
Since the mass of a point is constant, and its acceleration, the equation expressing the basic law of dynamics can be represented as
The equation simultaneously expresses the theorem on the change in the momentum of a point in differential form: time derivative of the momentum of a point is equal to the geometric sum of the forces acting on the point.
Let's integrate this equation. Let the mass point m, moving under the action of a force (Fig. 15), has at the moment t\u003d 0 speed, and at the moment t 1 - speed.
Fig.15
Let us then multiply both sides of the equality by and take definite integrals from them. In this case, on the right, where the integration is over time, the limits of the integrals will be 0 and t 1 , and on the left, where the speed is integrated, the limits of the integral will be the corresponding values of the speed and
. Since the integral of 
equals ,
then as a result we get:
.
The integrals on the right are the impulses active forces. So we end up with:
.
The equation expresses the theorem on the change in the momentum of a point in the final form: the change in the momentum of a point over a certain period of time is equal to the geometric sum of the impulses of all forces acting on the point over the same period of time ( rice. 15).
When solving problems, instead of a vector equation, equations in projections are often used.
In the case of rectilinear motion along the axis Oh the theorem is expressed by the first of these equations.
Example 9 Find the law of motion material point masses m moving along the axis X under the action of a force constant in modulus F(Fig. 16) under initial conditions: , at 
.
Fig.16
Solution. Let us compose a differential equation of motion of a point in the projection onto the axis X: . Integrating this equation, we find: 
. The constant is determined from the initial condition for the velocity and is equal to . Finally
.
Further, taking into account that v = dx/dt, we arrive at the differential equation: 
, integrating which we get
The constant is determined from the initial condition for the coordinate of the point. She is equal. Therefore, the law of motion of a point has the form
Example 10. Load weight R(Fig. 17) begins to move from rest along a smooth horizontal plane under the action of a force F=kt. Find the law of motion of the load.
Fig.17
Solution. We choose the origin of the coordinate system O in the initial position of the load and direct the axle X in the direction of movement (Fig. 17). Then the initial conditions look like: x(t = 0) = 0,v( t = 0) = 0. Forces act on the load F,P and the reaction force of the plane N. The projections of these forces on the axis X matter Fx = F = kt, Rx = 0, N x= 0, so the corresponding equation of motion can be written as follows: . Separating the variables in this differential equation and then integrating, we get: v = gkt 2 /2P + C one . Substituting the initial data ( v(0) = 0), we find that C 1 = 0, and we get the law of speed change 
.
The last expression, in turn, is a differential equation, integrating which we will find the law of motion of a material point: 
. The constant entering here is determined from the second initial condition X(0) = 0. It is easy to see that . Finally
Example 11. On a load at rest on a horizontal smooth plane (see Fig. 17) at a distance a from the origin, begins to act in the positive direction of the axis x power F=k 2 (P/g)x, where R - cargo weight. Find the law of motion of the load.
Solution. The equation of motion of the considered load (material point) in the projection onto the axis X
The initial conditions of equation (1) have the form: x(t = 0) = a, v( t = 0) = 0.
We represent the time derivative of velocity entering equation (1) as follows:
.
Substituting this expression into equation (1) and reducing by ( P/g), we get
Separating the variables in the last equation, we find that . Integrating the latter, we have: . Using initial conditions 
, we get , and, therefore,
, 
. (2)
Since the force acts on the load in the positive direction of the axis X, it is clear that it must also move in the same direction. Therefore, in solution (2), the plus sign should be chosen. Replacing further in the second expression (2) with , we obtain a differential equation for determining the law of movement of the load. Whence, separating the variables, we have
.
Integrating the latter, we find: 
. After finding the constant, we finally get
Example 12. Ball M masses m(Fig.18) falls without initial velocity under the action of gravity. As the ball falls, it experiences resistance , where – constant drag coefficient. Find the law of motion of the ball.
Fig.18
Solution. Let us introduce a coordinate system with the origin at the point where the ball is located at t = 0, directing the axis at vertically down (Fig. 18). The differential equation of motion of the ball in the projection onto the axis at then has the form
The initial conditions for the ball are written as follows: y(t = 0) = 0, v( t = 0) = 0.
Separating variables in equation (1)
and integrating, we find: , where . Or after finding a constant
or . (2)
It follows from this that the limiting speed, i.e. speed at , is equal to .
To find the law of motion, we replace v in equation (2) by dy/dt. Then, integrating the resulting equation with allowance for the initial condition, we finally find
.
Example 13 Research submarine of spherical shape and mass m= = 1.5×10 5 kg starts to sink with the engines off, having a horizontal speed v X 0 = 30 m/s and negative buoyancy R 1 = 0.01mg, where 
is the vector sum of the Archimedean buoyancy force Q and gravity mg acting on the boat (Fig. 20). Water resistance force 
, kg/s. Determine the equations of motion of the boat and its trajectory.
General theorems of the dynamics of a system of bodies. Theorems on the motion of the center of mass, on the change in the momentum, on the change in the main moment of the momentum, on the change in kinetic energy. Principles of d'Alembert, and possible displacements. General equation of dynamics. Lagrange's equations.
ContentThe work done by the force, is equal to the scalar product of the force vectors and the infinitesimal displacement of the point of its application :
,
that is, the product of the modules of the vectors F and ds and the cosine of the angle between them.
The work done by the moment of force, is equal to the scalar product of the vectors of the moment and the infinitesimal angle of rotation :
.
d'Alembert principle
The essence of d'Alembert's principle is to reduce the problems of dynamics to the problems of statics. To do this, it is assumed (or it is known in advance) that the bodies of the system have certain (angular) accelerations. Next, the forces of inertia and (or) moments of inertia forces are introduced, which are equal in magnitude and reciprocal in direction to the forces and moments of forces, which, according to the laws of mechanics, would create given accelerations or angular accelerations
Consider an example. The body makes a translational motion and external forces act on it. Further, we assume that these forces create an acceleration of the center of mass of the system . According to the theorem on the movement of the center of mass, the center of mass of a body would have the same acceleration if a force acted on the body. Next, we introduce the force of inertia:
.
After that, the task of dynamics is:
.
;
.
For rotational movement proceed in a similar way. Let the body rotate around the z axis and external moments of forces M e zk act on it. We guess these moments create angular accelerationεz . Next, we introduce the moment of inertia forces M И = - J z ε z . After that, the task of dynamics is:
.
Turns into a static task:
;
.
The principle of possible movements
The principle of possible displacements is used to solve problems of statics. In some problems, it gives a shorter solution than writing equilibrium equations. This is especially true for systems with connections (for example, systems of bodies connected by threads and blocks), consisting of many bodies
The principle of possible movements.
For the equilibrium of a mechanical system with ideal constraints, it is necessary and sufficient that the sum of the elementary works of all active forces acting on it for any possible relocation system was zero.
Possible system relocation- this is a small displacement, at which the connections imposed on the system are not broken.
Perfect Connections- these are bonds that do not do work when the system is moved. More precisely, the sum of work performed by the links themselves when moving the system is zero.
General equation of dynamics (d'Alembert - Lagrange principle)
The d'Alembert-Lagrange principle is a combination of the d'Alembert principle with the principle of possible displacements. That is, when solving the problem of dynamics, we introduce the forces of inertia and reduce the problem to the problem of statics, which we solve using the principle of possible displacements.
d'Alembert-Lagrange principle.
When a mechanical system moves with ideal constraints at each moment of time, the sum of the elementary works of all applied active forces and all inertia forces on any possible displacement of the system is equal to zero:
.
This equation is called general equation of dynamics.
Lagrange equations
Generalized coordinates q 1 , q 2 , ..., q n is a set of n values that uniquely determine the position of the system.
The number of generalized coordinates n coincides with the number of degrees of freedom of the system.
Generalized speeds are the derivatives of the generalized coordinates with respect to time t.
Generalized forces Q 1 , Q 2 , ..., Q n
.
Consider a possible displacement of the system, in which the coordinate q k will receive a displacement δq k . The rest of the coordinates remain unchanged. Let δA k be the work done by external forces during such a displacement. Then
δA k = Q k δq k , or
.
If, with a possible displacement of the system, all coordinates change, then the work done by external forces during such a displacement has the form:
δA = Q 1 δq 1 + Q 2 δq 2 + ... + Q n δq n.
Then the generalized forces are partial derivatives of the displacement work:
.
For potential forces with potential Π,
.
Lagrange equations are the equations of motion of a mechanical system in generalized coordinates:
Here T - kinetic energy. It is a function of generalized coordinates, velocities, and possibly time. Therefore, its partial derivative is also a function of generalized coordinates, velocities, and time. Next, you need to take into account that the coordinates and velocities are functions of time. Therefore, to find the total time derivative, you need to apply the rule of differentiation of a complex function:
.
References:
S. M. Targ, Short Course theoretical mechanics, graduate School", 2010.
Similarly, as for one material point, we derive a theorem on the change in the momentum for the system in various forms.
We transform the equation (theorem on the movement of the center of mass of a mechanical system)
in the following way:
;
;
The resulting equation expresses the theorem on the change in the momentum of a mechanical system in differential form: the time derivative of the momentum of a mechanical system is equal to the main vector of external forces acting on the system .
In projections onto Cartesian coordinate axes:
; 
; 
.
Taking the integrals of both parts of the last equations in time, we obtain a theorem on the change in the momentum of a mechanical system in integral form: the change in the momentum of a mechanical system is equal to the momentum of the main vector of external forces acting on the system .
.
Or in projections onto the Cartesian coordinate axes:
; 
; 
.
Consequences from the theorem (laws of conservation of momentum)
The law of conservation of momentum is obtained as special cases of the theorem on the change in momentum for a system depending on the features of the system of external forces. Internal forces can be anything, as they do not affect changes in momentum.
Two cases are possible:
1. If the vector sum of all external forces applied to the system is equal to zero, then the momentum of the system is constant in magnitude and direction
2. If the projection of the main vector of external forces on any coordinate axis and/or and/or is equal to zero, then the projection of the amount of motion on the same axes is a constant value, i.e. and/or and/or respectively.
Similar records can be made for a material point and for a material point.
The task. From a gun whose mass M, a projectile of mass flies out in a horizontal direction m with speed v. Find speed V guns after firing.
Solution. All external forces acting on the gun-projectile mechanical system are vertical. Hence, based on the corollary of the theorem on the change in the momentum of the system, we have: .
The amount of movement of the mechanical system before the shot:
The amount of movement of the mechanical system after the shot:
.
Equating the right parts of the expressions, we get that
.
The “-” sign in the resulting formula indicates that after the shot, the gun will roll back in the direction opposite to the axis Ox.
EXAMPLE 2. A jet of liquid with a density flows out at a speed V from a pipe with a cross-sectional area F and hits a vertical wall at an angle. Determine the fluid pressure on the wall.
SOLUTION. We apply the theorem on the change in momentum in integral form to the volume of liquid with mass m hitting a wall over a period of time t.
MESHCHERSKY EQUATION
(basic equation of the dynamics of a body of variable mass)
In modern technology, cases arise when the mass of a point and a system does not remain constant in the process of movement, but changes. So, for example, during the flight of space rockets, due to the ejection of combustion products and individual unnecessary parts of rockets, the change in mass reaches 90-95% of the total initial value. But not only space technology can be an example of the dynamics of the movement of a variable mass. In the textile industry, there is a significant change in the mass of various spindles, spools, rolls at modern machine and machine speeds.
Consider the main features associated with a change in mass, using the example of the translational motion of a body of variable mass. The basic law of dynamics cannot be directly applied to a body of variable mass. Therefore, we get differential equations motion of a point of variable mass, applying the theorem on the change in the momentum of the system.
Let a point of mass m+dm moves at speed. Then there is a detachment from the point of some particle with a mass dm moving at speed.
The amount of motion of the body before the detachment of the particle:
The amount of motion of a system consisting of a body and a detached particle after its detachment:
Then the change in momentum is:
Based on the theorem on the change in the momentum of the system:
Let us denote the value - the relative velocity of the particle:
Denote 
the value R called reactive force. The jet force is the thrust of the engine, due to the release of gas from the nozzle.
Finally we get
-
This formula expresses the basic equation of the dynamics of a body of variable mass (Meshchersky's formula). It follows from the last formula that the differential equations of motion of a point of variable mass have the same form as for a point of constant mass, except for the additional reactive force applied to the point due to the change in mass.
The basic equation of the dynamics of a body of variable mass indicates that the acceleration of this body is formed not only due to external forces, but also due to the reactive force.
Reactive force is a force akin to that felt by a shooting person - when firing a pistol, it is felt by the hand; when shooting from a rifle, it is perceived by the shoulder.
Tsiolkovsky's first formula (for a single-stage rocket)
Let a point of variable mass or a rocket move in a straight line under the action of only one reactive force. Since for many modern jet engines , where is the maximum reactive force allowed by the engine design (engine thrust); is the force of gravity acting on the engine earth's surface. Those. the foregoing allows the component in the Meshchersky equation to be neglected and for further analysis to accept this equation in the form: ,
Denote:
Fuel reserve (for liquid-propellant jet engines - the dry mass of the rocket (its remaining mass after all the fuel burns out);
The mass of particles separated from the rocket; considered as a variable varying from to .
Let us write the equation of rectilinear motion of a point of variable mass in the following form:
Since the formula for determining the variable mass of a rocket
Therefore, the equations of motion of a point 
Taking the integrals of both parts, we get
where - characteristic speed- this is the speed that the rocket acquires under the action of thrust after the eruption of all particles from the rocket (with liquid-propellant jet engines - after burning out all the fuel).
Taken out of the integral sign (which can be done on the basis of the mean value theorem known from higher mathematics) is the average velocity of particles ejected from the rocket.
Quantity of system movement call the geometric sum of the quantities of motion of all material points of the system
To clarify the physical meaning of (70), we calculate the derivative of (64)
.
(71)
Solving (70) and (71) together, we obtain
.
(72)
In this way, the momentum vector of a mechanical system is determined by the product of the mass of the system and the velocity of its center of mass.
Let us calculate the derivative of (72)
.
(73)
Solving (73) and (67) together, we obtain
.
(74)
Equation (74) expresses the following theorem.
Theorem: The time derivative of the momentum vector of the system is equal to the geometric sum of all external forces of the system.
When solving problems, equation (74) must be projected onto the coordinate axes:
.
(75)
Analysis of (74) and (75) implies the following law of conservation of momentum of the system: If the sum of all forces of the system is equal to zero, then its momentum vector retains its magnitude and direction.
If 
, then 
,Q
=
const
.
(76)
In a particular case, this law can be fulfilled along one of the coordinate axes.
If 
, then, Q z =
const.
(77)
It is advisable to use the momentum change theorem in cases where liquid and gaseous bodies enter the system.
Theorem on the change in the angular momentum of a mechanical system
The amount of motion characterizes only the translational component of the motion. To characterize the rotational motion of a body, the concept of the main moment of the quantities of motion of the system relative to a given center (kinetic moment) is introduced.
The momentum of the system relative to a given center is the geometric sum of the moments of the quantities of motion of all its points relative to the same center
.
(78)
By projecting (22) on the coordinate axes, one can obtain the expression for the angular momentum with respect to the coordinate axes
.
(79)
The angular momentum of the body about the axes is equal to the product of the moment of inertia of the body about this axis by the angular velocity of the body
.
(80)
From (80) it follows that the kinetic moment characterizes only the rotational component of the motion.
A characteristic of the rotational action of a force is its moment relative to the axis of rotation.
The momentum change theorem establishes the relationship between the characteristic of rotational motion and the force that causes this motion.
Theorem: The time derivative of the angular momentum vector of the system with respect to some center is equal to the geometric sum of the moments of all external forces of the system with respect tothe same center
.
(81)
When solving engineering problems (81), it is necessary to project onto the coordinate axes
Their analysis (81) and (82) implies momentum conservation law: If the sum of the moments of all external forces about the center (or axis) is equal to zero, then the kinetic moment of the system about this center (or axis) retains its magnitude and direction.
,
or 
The angular momentum cannot be changed by the action of the internal forces of the system, but due to these forces it is possible to change the moment of inertia, and hence the angular velocity.
- In contact with 0
- Google+ 0
- OK 0
- Facebook 0
