Many elements of building structures (columns, racks, supports) are under the influence of compressive forces applied not in the center of gravity of the section. On fig. 12.9 shows the column on which the floor beam rests. As you can see, the force acts with respect to the axis of the column with an eccentricity e, and thus, in an arbitrary section ah columns along with longitudinal force N = -R there is a bending moment, the magnitude of which is equal to Re. Eccentric tension (compression) of the rod is a type of deformation in which the resultant external forces act along a straight line parallel to the axis of the rod. In what follows, we will mainly consider problems of eccentric compression. In case of eccentric tension, in all the calculation formulas given, the sign in front of the force should be changed R to the opposite.
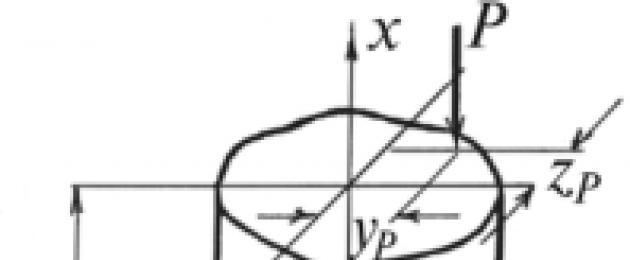
Let a rod of arbitrary cross section (Fig. 12.10) be loaded at the end with an eccentrically applied compressive force R, directed parallel to the axis Oh. Accept positive

directions of the main axes of inertia of the section OU and Oz so that the point of application of force R was in the first quarter of the coordinate axes. We denote the coordinates of the point of application of force R through y r and z P -
Internal forces in an arbitrary section of the rod are equal to
The minus signs of the bending moments are due to the fact that in the first quarter of the coordinate axes these moments cause compression. Quantities internal efforts in this example, do not change along the length of the rod, and thus, the distribution of stresses in sections sufficiently remote from the place of application of the load will be the same.
Substituting (12.11) into (12.1), we obtain the formula for normal stresses under eccentric compression:

This formula can be converted to the form

where i , i- main radii of inertia of the section. Wherein
Putting in (12.12) o = 0, we obtain the equation zero line:
Here at 0 and z 0 - coordinates of points of the zero line (Fig. 12.11). Equation (12.14) is the equation of a straight line that does not pass through the center of gravity of the section. To draw a zero line, we find the points of its intersection with the coordinate axes. Assuming in (12.14) successively y 0 = 0 and z0= 0, respectively, we find
where a z and and y- segments cut off by the zero line on the coordinate axes (Fig. 12.11).


Let us establish the features of the position of the zero line under eccentric compression.
- 1. It follows from formulas (12.15) that and at and a z have opposite signs respectively y r and z P - Thus, the zero line passes through those quarters of the coordinate axes that do not contain the point of application of the force (Fig. 12.12).
- 2. As the point of application of force approaches R in a straight line to the center of gravity of the section, the coordinates of this point y r and z P decrease. From (12.15) it follows that in this case the absolute values of the lengths of the segments and at and a z increase, that is, the zero line moves away from the center of gravity, remaining parallel to itself (Fig. 12.13). In the limit at Z P = y P = 0 (force is applied at the center of gravity) the zero line is removed to infinity. In this case, the stresses in the cross section will be constant and equal to o = -P/F.
- 3. If the point of application of force R located on one of the main axes, the zero line is parallel to the other axis. Indeed, putting in (12.15), for example, y r= 0, we get that and at= that is, the zero line does not cross the axis OU(Fig. 12.14).
- 4. If the point of application of the force moves along a straight line that does not pass through the center of gravity, then the zero line rotates around a certain point. Let's prove this property. Points of application of forces R x and R 2, located on the coordinate axes correspond to the zero lines 1 - 1 and 2-2, parallel to the axes (Fig. 12.15), which intersect at the point D. Since this point belongs to two zero lines, the stresses at this point from simultaneously applied forces R x and R 2 will be equal to zero. Since any force R 3 , the point of application of which is located on a straight line R ( R 2 , can



decompose into two parallel components applied at the points Pj and R 2, then it follows that the stresses at the point D from the force R 3 are also equal to zero. Thus, the zero line 3-3, corresponding to the strength R 3 , passes through a point D.
In other words, a set of points R, located on a straight line R ( R 2 , corresponds to a pencil of lines passing through a point D. The converse statement is also true: when the zero line rotates around a certain point, the point of application of the force moves along a straight line that does not pass through the center of gravity.
If the zero line crosses the section, then it divides it into zones of compression and tension. As in oblique bending, it follows from the flat section hypothesis that the stresses reach their greatest values at the points farthest from the zero line. The nature of the stress diagram in this case is shown in Fig. 12.16, a.
If the zero line is located outside the section, then at all points of the section the stresses will be of the same sign (Fig. 12.16, b).
Example 12.3. Let us construct a diagram of normal stresses in an arbitrary section of an eccentrically compressed rectangular column with dimensions b X h(Fig. 12.17). The squares of the radii of inertia of the section according to (12.22) are 

The segments cut off by the zero line on the coordinate axes are determined by the formulas (12.15):

Substituting sequentially into (12.12) the coordinates of the points C and the most distant from the zero line AT(Fig. 12.18)
find 


Plot o is shown in fig. 12.18. The highest compressive stresses in absolute value are four times higher than the stresses that would be in the case of a central application of force. In addition, significant tensile stresses appeared in the cross section. Note that from (12.12) it follows that at the center of gravity (y = z\u003d 0) the stresses are equal o \u003d -P/F.
Example 12.4. Cutout strip loaded with tensile force R(Fig. 12.19, a). Compare the stresses in the section lv, far enough from the end and the place of the cut, with stresses in the section CD at the cutout.
in section AB(Fig. 12.19, b) strength R causes central tension and the stresses are a = P/F = P/bh.

in section CD(Fig. 12.19, in) line of force R does not pass through the center of gravity of the section, and therefore eccentric tension occurs. By changing the sign in formula (12.12) to the opposite and taking y r= 0, we obtain for this section
Taking 

Zero line in section CD parallel to axis OU and crosses the axis Oz on distance a =-i 2 y /z P- b/ 12. At the points of the section furthest from the zero line C(z - -b/ 4) and D(z - b/ 4) stresses according to (12.16) are equal

Diagrams of normal stresses for sections LV and CD shown in fig. 12.19, b, c.
Thus, despite the fact that the cross section CD has an area two times smaller than the cross section AB, due to the eccentric application of the force, the tensile stresses in the weakened section increase not by a factor of two, but by a factor of eight. In addition, significant compressive stresses appear in this section.
It should be noted that the above calculation does not take into account additional local stresses that occur near point C due to the presence of a recess. These stresses depend on the radius of the undercut (they increase with decreasing radius) and can significantly exceed the value found a c = 8P/bh. In this case, the nature of the stress diagram near point C will differ significantly from the linear one. The definition of local stresses (stress concentration) is discussed in Chapter 18.
Many building materials (concrete, brickwork, etc.) do not resist stretching well. Their tensile strength is many times less than that of compression. Therefore, the appearance of tensile stresses in structural elements made of such materials is undesirable. For this condition to be satisfied, it is necessary that the zero line is outside the section. Otherwise, the zero line will cross the section and tensile stresses will appear in it. If the zero line is tangent to the section contour, then the corresponding position of the force application point is the limit. In accordance with property 2 of the zero line, if the point of application of force approaches the center of gravity of the section, the zero line will move away from it. The locus of limit points corresponding to different tangents to the section contour is the boundary section kernels. The core of the section is a convex area around the center of gravity, which has the following property: if the point of application of the force is inside or on the border of this area, then at all points of the section the stresses have the same sign. The core of the section is a convex figure, since the zero lines must touch the envelope of the section contour and not cross it.
Through the dot BUT(Fig. 12.20) you can draw an infinite number of tangents (zero lines); while only tangent AC is tangent to the envelope, and a certain point of the section core contour must correspond to it. At the same time, for example, it is impossible to draw a tangent to the segment AB section contour because it intersects the section.
Let's build a section kernel for a rectangle (Fig. 12.21). For tangent 1 - 1 a 7 - b/ 2; a= . From (12.15) we find for point 1 corresponding to this tangent, z P \u003d -i 2 y / a 7 \u003d -b / 6; y r - 0. For tangent 2-2 and y - k / 2; a 7 \u003d ° °, and the coordinates of point 2 will be equal atR- -h/6; z P - 0. According to property 4 of the zero line, the points of application of the force corresponding to various tangents to the lower right corner point of the section are located on the straight line 1-2. The position of points 3 and 4 is determined from the symmetry conditions. Thus, the section kernel for a rectangle is a rhombus with diagonals b/3 and FROM.


To build a section kernel for a circle, it is enough to draw one tangent (Fig. 12.22). Wherein a = R; a= °o.
"U U ^^
Considering that for a circle i y - J y /F - R / 4, from (12.15) we get 
Thus, the section kernel for a circle is a circle with a radius R/4.
On fig. 12.23, a, 6 section cores for an I-beam and a channel are shown. The presence of four corner points of the core of the section in each of these examples is due to the fact that the envelope of the contour for both the I-beam and the channel is a rectangle.


Eccentric tension (compression) is caused by a force parallel to the beam axis, but not coinciding with it (Fig. 9.4).
The projection of the point of application of force on the cross section is called the pole or power point, and the straight line passing through the pole and the center of the section is called the line of force.
Eccentric tension (compression) can be reduced to axial tension (compression) and oblique bending if the force P is transferred to the center of gravity of the section. So, the force P, marked in Fig. 9.4 with one dash G will cause axial tension of the beam, and a pair of forces marked with two dashes will cause an oblique bend.
Based on the principle of independence of the action of stress forces at the points of the cross section during eccentric tension (compression) are determined by the formula
In this formula, the axial force, bending moments, as well as the coordinates of the section point at which the stress is determined, must be substituted with their signs. For bending moments, we will take the same sign rule as in oblique bending, and we will consider the axial force positive when it causes tension.
If the coordinates of the pole are denoted by , then the moment Formula (9.5) takes the form
![]()
It can be seen from this equation that the ends of the stress vectors at the points of the section are located on the plane. The line of intersection of the stress plane with the plane of the cross section is a neutral line, the equation of which is found by equating right side equality (9.6) to zero. After reduction by P we get
![]()
![]()
Thus, the neutral line in off-center tension (compression) does not pass through the center of gravity of the section and is not perpendicular to the plane of action of the bending moment. The neutral line cuts off segments on the coordinate axes
We represent the moments of inertia as the product of the cross-sectional area and the square of the corresponding radius of inertia
Then expressions (9.8) can be written as follows:
![]()
From formulas (9.8) it can be seen that the pole and the neutral line are always located on opposite sides of the center of gravity of the section, and the position of the neutral line is determined by the coordinates of the pole.


When the pole approaches the center of gravity of the section along the line of force, the neutral line will move away from the center, remaining parallel to its original direction. In the limit at , the neutral line recedes to infinity. In this case, there will be a central tension (compression) of the beam.
On the line of force, you can always find such a position of the pole, in which the neutral line will touch the contour of the section, without crossing it anywhere. If we draw all possible neutral lines so that they touch the contour of the section, without crossing it anywhere, and find the poles corresponding to them, then it turns out that the poles will be located on a closed line that is quite specific for each section. The area bounded by this line is called the core of the section. In a circular section, for example, the core is a circle with a diameter 4 times smaller than the diameter of the section, and in rectangular and I-sections, the core has the shape of a parallelogram (Fig. 9.5).
It follows from the very construction of the core of the section that as long as the pole is inside the core, the neutral line will not cross the contour of the section and the stresses in the entire section will be of the same sign. If, however, the pole is located outside the core, then the neutral line will cross the contour of the section, and then stresses of different signs will act in the section. This circumstance must be taken into account when calculating off-center compression of racks made of brittle materials. Since brittle materials poorly perceive tensile loads, it is desirable to apply external forces to the rack so that only compressive stresses act in the entire section. To do this, the point of application of the resultant of external forces compressing the rack must be inside the core of the section.
The calculation for strength in off-center tension and compression is carried out in the same way as in oblique bending - according to the stress at the dangerous point of the cross section. Dangerous is the point of the section, the most distant from its neutral line. However, in cases where compressive stress acts at this point, and the strut material is brittle, the point where the greatest tensile stress acts may be dangerous.
The stress diagram is built on an axis perpendicular to the neutral line of the section, and is limited by a straight line (see Fig. 9.4).
The strength condition is written as follows.
Rice. 12.3. Eccentric tension of the beam
Stresses at an arbitrary point of the section with coordinates (x, y) based on the principle of independence of the action of forces can be calculated as follows (algebraic sum)
Their equation (12.4) implies that the stress diagram in the considered section forms a plane. The equation of the neutral line, at the points of which the normal stresses are equal to zero, we obtain from (12.4), equating the expression to zero, i.e.
 (12.5)
(12.5)
It follows from the resulting equation that the neutral line does not pass through the center of gravity of the section, which coincides with the origin. In addition, if the coordinates of the force application point (x 0, y 0) are positive, then according to at least one of the x or y coordinates of equation (12.4) must be negative and therefore, if the point of application of the force is in the first quadrant, then the neutral line must pass through quadrants 2,3 and 4 (Fig. 12.4).
It is known (analytical geometry) that if a straight line is given by an equation of the form

then the distance from the origin of coordinates to the line will be equal to

In the case under consideration (12.5), we obtain (Fig. 12.4)
 (12.5a)
(12.5a)
It follows from the obtained expression that when the point of application of the force P approaches the center of gravity of the section, i.e. as the value of the coordinates x 0 , y 0 decreases, the distance ρ from the center of gravity of the section to the neutral line increases.
| σC |
| x |
| y |
| BUT |
Fig.12.4. Stress distribution in eccentric tension
In the limit at x 0 =y 0 =0, i.e. when the force P is applied at the center of gravity of the section, the neutral line is at infinity. In this case, a simple (central) tension or compression takes place, all stresses in the cross section are of the same sign and are equal to each other.
If the neutral line crosses the section, then on one side of it a tension zone appears, and on the other, a compression zone (Fig. 12.4). By drawing lines parallel to the neutral line and tangent to the contour of the section, one can find the most distant points from the neutral line at which the normal stresses reach their maximum values. In the considered case, these are points C and D.
We write the strength conditions at these points in the form
where x C , y C , x D , y D are coordinates of dangerous points. The signs of the terms in formulas (12.6) are chosen based on the analysis of the directions of action of the bending moments and the normal force. If the neutral line does not cross the cross section, then all normal stresses will be of the same sign.
The area in the vicinity of the center of gravity of the section, which has the property that when a force P is applied within this area, the stresses at all points of the section will be of the same sign, is called section kernel.
Some materials (concrete, brick, gray cast iron) resist tension much worse than compression. For appropriate structures, it is important that tensile stresses do not occur in the material, which means that compressive forces must be applied within the core of the section.
If the force in eccentric tension (compression) is applied at the boundary of the core of the section, then the neutral line touches the contour of the section. This condition is used to determine the dimensions of the section core. For example, for a bar with a circular cross section, it follows from the condition of geometric symmetry that the core of the section should have the shape of a circle (Fig. 12.5). Let the point of application of the force Р be located on the axis Oy at a distance from the origin equal to r (the coordinates of the point of application of the force are x 0 =0, y 0 =r). The neutral line equation in this case takes the form (see formula 12.5)

This is the equation of a straight parallel axis Ox. Since the core of the section is a circle of radius r, the neutral line must touch the contour at point A (Fig. 12.5). The distance from the origin of coordinates and the neutral line is equal to the radius of the circumference of the cross section of the beam R. Then, taking into account the expression (12.5a), we find

Hence r=R/4, i.e. the core of a beam of circular cross section with a radius R is a circle with a radius R/4.
Eccentric compression. Building section kernels. Bending with twist. Calculations for strength under complex stress state.
Off-center compression is a type of deformation in which the longitudinal force in the cross section of the rod is not applied at the center of gravity. At eccentric compression, in addition to the longitudinal force (N), there are two bending moments ( and ).
It is considered that the rod has a high bending rigidity in order to neglect the deflection of the rod under eccentric compression.
Let's transform the formula of moments for eccentric compression , substituting the values of bending moments: .
Let us denote the coordinates of a certain point of the zero line under eccentric compression , and substitute them into the formula for normal stresses under eccentric compression. Considering that the stresses at the points of the zero line are equal to zero, after the reduction by , we obtain the equation of the zero line for eccentric compression: ![]() .
.
The zero line for eccentric compression and the point of application of the load are always located on opposite sides of the center of gravity of the section.

The segments cut off by the zero line from the coordinate axes, denoted by and , can be easily found from the zero line equation for eccentric compression. If we first accept ![]() and then accept
and then accept ![]() , then we find the points of intersection of the zero line under eccentric compression with the principal central axes:
, then we find the points of intersection of the zero line under eccentric compression with the principal central axes:

Zero line under eccentric compression will divide the cross section into two parts. In one part, the stresses will be compressive, in the other - tensile. The strength calculation, as in the case of oblique bending, is carried out according to the normal stresses that occur at the dangerous point of the cross section (the furthest from the zero line). 
Section core - a small area around the center of gravity of the cross section, characterized by the fact that any compressive longitudinal force applied inside the core causes compressive stresses at all points of the cross section.
Examples of the section kernel for rectangular and circular bar cross sections.

Bending with twist. Shafts of machines and mechanisms are often subject to such loading (simultaneous action of torques and bending moments). To calculate the beam, it is necessary first of all to establish dangerous sections. To do this, plots of bending and torque moments are built.
Using the principle of independence of the action of forces, we determine the stresses that arise in the beam separately for torsion and for bending.
During torsion in the cross sections of the beam, shear stresses arise, reaching the highest value at the points of the section contour  When bending in the cross sections of the beam, normal stresses arise, reaching the highest value in the extreme fibers of the beam
When bending in the cross sections of the beam, normal stresses arise, reaching the highest value in the extreme fibers of the beam  .
.
Consider a straight rod loaded at the end with forces directed parallel to the axis Oh. The resultant of these forces F applied at the point FROM. In the local right-handed coordinate system yOz, coinciding with the main central axes of the section, the coordinates of the point FROM equal a and b(Fig. 5.18).
Let us replace the applied load with a statically equivalent system of forces and moments. To do this, we transfer the resultant force F to the center of gravity of the section O and load the rod with two bending moments equal to the product of the force T^ on its arms with respect to the coordinate axes: M ff = Fa and Mz = Fb.
Note that according to the rule of the right-handed coordinate system for the point C, which lies in the first quadrant, the bending moments will formally receive the following
Rice. 5.18.Straight rod loaded at the end with forces directed parallel to the axisOh
blowing signs: M y \u003d Fa and M 7 = -Fb. In this case, in the elementary area lying in the first quarter, both moments cause tensile stress.
Using the principle of independence of the action of forces, we determine the stresses at the current point of the section with coordinates at and z from each power factor separately. The total voltage is obtained by summing all three voltage components:
Let us determine the position of the neutral axis. To do this, in accordance with formula (5.69), we equate to zero the value of the normal stress at the current point:

As a result of simple transformations, we obtain the neutral line equation
where i y and i z - main radii of inertia determined by formulas (3.14).
Thus, in the case of eccentric tension-compression, the neutral line does not pass through the center of gravity of the section (Fig. 5.19), as indicated by the presence in equation (5.70) of a free term that differs from zero.
The maximum stresses occur at the points of the section BUT and AT, farthest from the neutral line. Let's establish the relationship between the coordinates of the force application point and the position of the neutral line. To do this, we determine the points of intersection of the coordinate axes by this line:

Rice. 5.19.
The resulting formulas show that the coordinate of the force application point a and the coordinate of the point of intersection of the neutral line of the coordinate axis Oz(point r 0) have opposite signs. The same can be said about the quantities b and at 0 . Thus, the point of application of the resultant force and the neutral line are on opposite sides of the origin.
According to the formulas obtained, as the point of application of force approaches the center of gravity of the section, the neutral line moves away from central zone. In the limiting case (a = b = 0) we arrive at the case of central tension-compression.
It is of interest to determine the zone of force application, in which the stresses in the section will have the same sign. In particular, for materials that poorly resist stretching, it is rational to apply a compressive force precisely in this zone, so that only compressive stresses act in the section. Such a zone around the center of gravity of the section is called section core.
If the force is applied at the core of the section, then the neutral line does not intersect the section. If a force is applied along the boundary of the section core, the neutral line touches the section contour. Formula (5.71) can be used to determine the core of the section.
If we represent the neutral line as a tangent to the contour of the section and consider all possible positions of the tangent and the points of application of the force corresponding to these positions, then the points of application of the force will outline the core of the section.

Rice. 5.20.
a - ellipse; 6 - rectangle
- In contact with 0
- Google Plus 0
- OK 0
- Facebook 0